General Density Equations
From time to time, it will be necessary to adjust the density of a clear brine completion fluid. A completion fluid may become diluted by rain, seawater, or by water from the producing formation. Additionally, bottomhole pressure conditions may demand that a fluid weight up be performed to maintain well control. On the other hand, cutting back a fluid's density may also be required to reduce invasion of wellbore fluids into the formation.
The most basic form of the density equation is given as mass per unit volume, ordinarily in units of pounds per gallon (lb/gal).
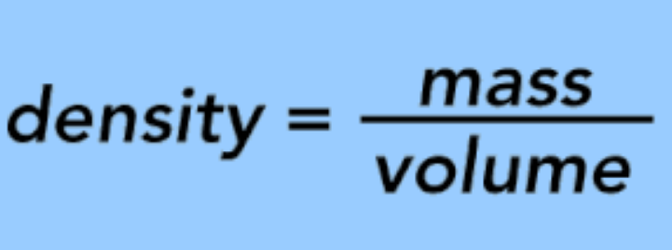
The equation can be rearranged to solve for weight, as shown here.

All density adjustment calculations are made using expanded forms of the preceding equations. Equation 7 is used for weight up or cutback calculations. It is the most general form used in most oilfield density adjustment calculations. Equation 8 states that the final volume is the combination of the starting volume and the added volume. A small error results from the complex interaction of water and the brine chemicals. These equations are good approximations, but they will require a field check for final density. Virtually all density calculations—weight up, cutback, or volume—are done using some variation of Equation 7 and/or Equation 8.
EQUATION 7.
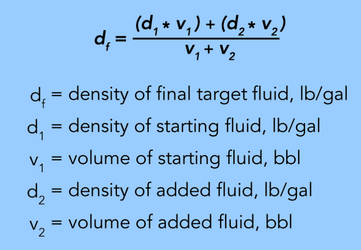
EQUATION 8.

The next group of equations deals with density adjustment, rearranging the terms in Equation 7 and Equation 8 to allow you to find the quantities you will need.
Mixing Two Known Fluids—Unknown Final Density
The least complicated situation involves determining the final density when mixing together two fluids of known density and known volume. The density is determined using Equation 7 as it is written above. Also in this case, the final volume (vf) is the sum of the volume of the two fluids straight out of Equation 8.
EXAMPLE A. Determining Final Density, df, When d1, v1, d2, and v2 are Known

Mixing Two Known Fluids—Known Final Density
The following situation arises frequently and may even be the most common volume density calculation. You know the densities of the two starting fluids (d1 and d2) and the desired final density (df). What you want to know are the volumes of the two fluids (v1 and v2) that you need to mix to get one barrel of final density (df) fluid. Start by using the Equation 9 to find the volume of fluid 1 (v1).
EQUATION 9.

Then, set the final volume (vf) to 1.00 and subtract the calculated volume (v1) to get the volume of fluid 2 (v2) using Equation 8.
EXAMPLE B. Finding Volume Proportions of Two Known Fluids Needed to Make One Barrel of Known Density Fluid

Kill Weight Fluids
Completion fluid density is specifically designed to control well pressure; this being the case, most brines are kill weight fluids. Determining the appropriate density has been outlined in some detail in the section "Fluid Categories." In some instances, shut in bottomhole pressure (SIBP) may be substituted for anticipated bottomhole pressure (BHP), and any overbalance or underbalance will be included; however, the calculation process is essentially the same, using <ac:macro ac:name="> and <ac:macro ac:name=">.
EXAMPLE C. Kill Weight Fluid Density
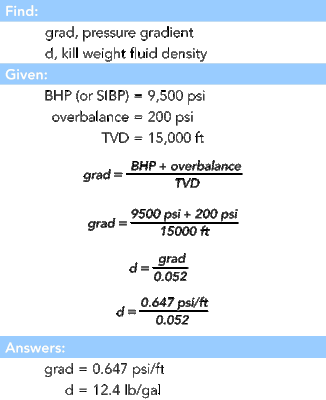
Typically, when using a completion fluid, the task will be to weight a fluid up to a higher density.
Weight Up of Working Fluid with Spike Fluid
The following calculations are applicable when the density of the circulating fluid needs to be increased. There are two cases. The first case, shown in Example D, uses Equation 7 to determine the density that can be achieved by adding a known amount of spike fluid to a known circulating volume. In this case, the final volume (vf) will increase. This case is identical to Example B of mixing two fluids of known density and volume. The final volume (vf) is the combined volume of initial fluid (v1) and spike fluid (v2).
EXAMPLE D. Weight Up with Spike Fluid

The second case, shown in Example E, is used when there is a volume limitation, meaning that the final volume (vf) is limited to the available holding capacity of the hole and surface equipment.
In this example, the volume is limited to the hole and surface equipment capacity (vf). The task is to find the maximum density that can be achieved with an initial starting fluid density (d1) using a fixed amount (v2) of spike fluid of a known density (d2).
EXAMPLE E. Weight Up Using Spike Fluid with Volume Limitation

Surface Density Correction
As a fluid circulates through a well, it experiences changes in temperature, and expands and contracts in relation to this heating and cooling. Because of this expansion and contraction, the density of the fluid may appear to be off. Fluid engineers will use one of the correction factors from Table 9 and apply Equation 10 to account for these temperature changes and determine the fluid's density at 60°F.
TABLE 9. Surface Density Correction Factors
| 8.4 - 9.0 lb/gal | 0.0002 |
| 9.1 - 11.0 lb/gal | 0.0003 |
| 11.1 - 14.5 lb/gal | 0.0004 |
| 14.6 - 16.0 lb/gal | 0.0005 |
| 16.1 - 18.0 lb/gal | 0.0006 |
| 18.1 - 19.2 lb/gal | 0.0007 |
EQUATION 10.
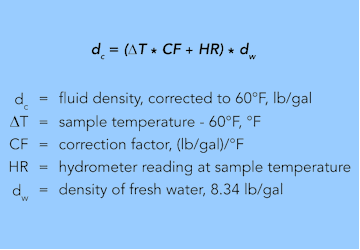
The example below illustrates a surface density correction for a fluid with an initial 10.0 lb/gal.
EXAMPLE F. Surface Density Correction
